Multicontact interface

A surface is never perfectly plane. This reality has probably been known for a very long time, maybe several thousands of years. In the eighteenth century, Coulomb attributed the cause of dry friction to the penetration of asperities in the antagonist surface.

Explanation of friction by Coulomb. Asperities interpenetrate so that the upper solid sliding requires asperities of lower solid to jump .

But the direct observation of the non flatness of surfaces is relatively recent. Modern mechanical and optical devices allow to explore surfaces from millimetric to nanometric scales. It can be seen that a surface is like mountains with summits and valleys. The only difference is that usually slopes of peaks are small, commonly about ten to tweenty percents. In the following figure the vertical scale is exaggerated so that it appears like sharp peaks.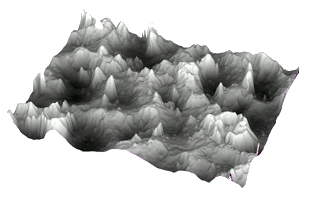

Surface of a metallic sample at the micrometric scale.

Now, when two solids are put into contact, we must imagine two moutain chains, one being turned upside down the other. Higher peaks touch the antagonist surfaces but most of valleys do not enter into contact. Two consequences appear as a result. First, the contact is rare. The actual contact area is usually a very small part of the nominal contact area. Second, the contact is multiple. It is composed of numerous spots randomly distributed on the surface.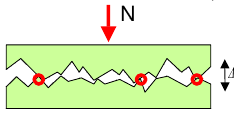

The actual contact between two solids is achieved by a large number of small spots. Left, asperities in contact between two rough surfaces. Right, view of contact between glass and elastomer, the contact spots are colored in red (full scale ~ 100 micrometers, from F. Deleau's thesis).

[1] C.A. Coulomb, Théorie des machines simples, Librairie Bachelier, Quai des Augustins, Paris, 1821, Reprint Albert Blanchard Paris, 2002.

[2] F. Deleau, D. Mazuyer, A. Koenen, Sliding friction at elastomer/glass contact: Influence of the wetting conditions and instability analysis, Tribology International, vol. 42, p. 149-159, 2009.